lightning36 asks :
I saw your Waffles vs Chad ROI comment. Is there an explanation for the math that even a former social sciences major can understand??
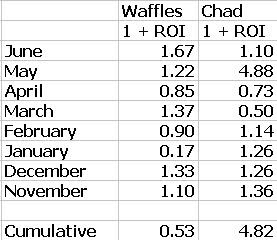
We multiply each month's (1+ROI) as seen below. As a point of reference, I experienced my last positive ROI month in March, 2004 (8.35%).




2 comments:
I disagree. In this context, I would say that what we poker players call ROI is not necessarily the same as the financial/statistical definition of ROI. The main reason for my statement is that 'poker ROI' is the the cumulative net of a series of independent, unrelated, finite investments (individual poker tournaments) and is not the compounding of a single investment over time (as in stock performance, etc.). I feel that we add, not multiply, to arrive at the total profit (or loss, lol) which then provides the 'ROI' ratio when compared with the sum of the buy-ins.
In this context, I feel that what is actually the most accurate calculation is, in effect, the simplest: total profit over 8 months divided by the sum of the 'investments' or tourney entry fees over the same time period. However, we only have the monthly ROI figures and we lack the monthly buy-in or profit figures, so we can't really come up with an accurate feel for total ROI over the eight months.
Lacking that data, if we assume equal buy-in totals for each months (and calculate the monthly P/L based on the ROI figures stated by Waffles in his post, we arrive at a total profit/buy-in, or 'ROI' of 7.63% for Waffles and 52.88% for Chad - which is the arithmetic mean.
Again, this is based on the premise that unlike a single investment increasing/decreasing in value over time, poker ROI is the net value of numerous short-term individual 'investments' we call tourneys.
I welcome your comments...
Thanks for your comments, doog. I agree that using ROI here is subject to interpretation and may differ from the other more traditional contexts. Nevertheless, I believe my model is valid given my assumptions :
(1) Each player has an equal, limited starting bankroll.
(2) For each particular time period (month), each player will fully utilize his bankroll. So for example, assume that there are $10, $20, $30 (and higher) buy-in tourneys and player A's bankroll at the beginning of the month is $3,000. For that particular month, he will either play 300 $10 tourneys, 150 $20 tourneys, 100 $30 tourneys, or a combination thereof. Note that winnings for the month are only applied for bankroll purposes to the next month.
Why should we hold back a good player and "assume equal buy-in totals for each month"? Or conversely, after a few months a bad player might not necessarily have the bankroll for the set amount of buy-ins.
However, you are right when you say that for this particular case "we only have the monthly ROI figures and we lack the monthly buy-in or profit figures, so we can't really come up with an accurate feel for total ROI over the eight months".
Post a Comment